NORTON'S THEOREM

Norton’s theorem was published in the year 1926 by Edward Lawry Norton, an American engineer at bell telephone laboratories. This theorem was published about 43 years after Thevenin’s theorem was published.

Norton’s theorem is similar or we can say dual of the Thevenin’s theorem. Briefly speaking, at one end where Thevenin’s theorem reduces a linear two terminal network to an equivalent constant voltage source and series resistance; Norton’s theorem reduces or replaces the network by an equivalent constant current source and a parallel resistance.

STATEMENT:

Norton’s theorem states that a linear two terminal circuit can be replaced by an equivalent circuit consisting of a current source I_N in parallel with a resistor R_N, where

I_N is the short circuit current through the terminals.

R_N is the equivalent resistance at the terminals when the independent sources are turned off.

HOW TO NORTONIZE A GIVEN CIRCUIT?

To illustrate this procedure, consider a simple circuit as follows:

Now follow the step by step procedure:

       1. Remove the resistance (if any) across the two given terminals and put a short circuit across them. In this case, there is no resistance at the terminals which follows from the diagram below.

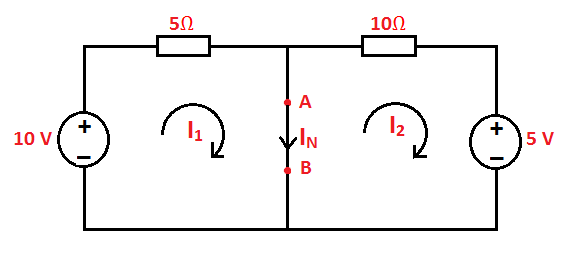
  2. Compute the short circuit current (I_SC) or in other words Norton current (I_N). Using the figure in step 1 above,

      ${{I}_{N}}={{I}_{1}}+{{I}_{2}}=\frac{10}{5}+\frac{5}{10}=2.5A$ 

      

  3. Remove all the independent voltage source by short circuit and retain their internal resistances if any. Similarly, remove all independent current sources and replace them by open circuits which are shown below.

 

Keep in mind that only independent sources need to be removed when calculating effective resistance while the dependent sources are left intact as they depend on other circuit variables.

When dependent sources are present, follow the same procedure as mentioned in the Thevenin’s theorem.

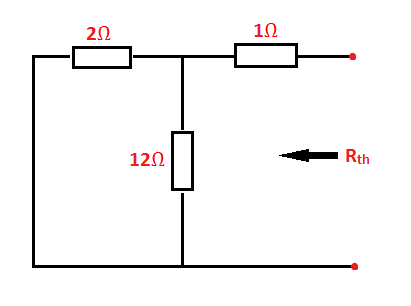
Thus, after turning off the independent sources R_eq comes out to be

${{R}_{eq}}={{R}_{N}}=5\Omega \parallel 10\Omega =\frac{5\times 10}{5+10}=\frac{10}{3}\Omega $ 

Note that, the procedure for finding the R_N is the same as R_th (Thevenin’s resistance) which implies that R_th = R_N.

 4. Now, connect the current source (I_N or I_SC) in parallel with Norton resistance to give Norton’s equivalent circuit.

 5. The load current I_L can be found using the current divider principle i.e.

      \[{{I}_{L}}=\left( \frac{{{R}_{N}}}{{{R}_{N}}+{{R}_{L}}} \right){{I}_{N}}\]

 

RELATIONSHIP BETWEEN THEVENIN’S AND NORTON’S EQUIVALENT CIRCUITS –

As we find R_N in the same way as R_th, therefore, R_N = R_th.

And ${{I}_{N}}=\frac{{{V}_{th}}}{{{R}_{th}}}\text{  or  }{{V}_{th}}={{R}_{N}}{{I}_{N}}$  

This equation is very useful as it can be applied to find the output impedance of a source. Practically, it is not possible to get inside a circuit and turn off internal sources. But we can measure open circuit output voltage and current that would flow when the output terminals are shorted.

THEVENIN'S THEOREM

Thevenin’s theorem was first proposed by a French telegraph engineer M.L.thevenin in 1883. Many a times we come across situations where we need to find the response (current, voltage, power) of a circuit against different values of load. Simplest example of this situation is in our homes; we connect different types of electrical devices (loads) to a single socket time and again. But then, what if we need to analyze the same circuit with different loads? It’s a tedious job you know!

WHAT THIS THEOREM DOES FOR US?

Thevenin’s theorem provides a mathematical technique for replacing a given network by a single voltage source with a series resistance, as viewed from the two output terminals.

STATEMENT:

Thevenin’s theorem states that a linear two terminal circuit can be replaced by an equivalent circuit consisting of a voltage source (V_th) in series with a resistor R_th, where

V_th – is the open circuit voltage as viewed from output terminals.

R_th – is the equivalent resistance at the terminals when the independent sources are turned off.

Thevenin’s theorem is a powerful tool in circuit analysis. It helps in replacing a large and complex circuit with a simple circuit consisting of voltage source and a series resistance.

HOW TO THEVENIZE A GIVEN CIRCUIT?

Consider the following circuit:

        1. Temporarily remove the load resistance R_L through which current is required.

        2. Compute the equivalent resistance as seen from the open terminals. Replace (turn off) independent voltage sources by short circuit and independent current sources by open circuit; and then calculate R_eq or R_th.
     

     NOTE: If the network has dependent sources, we turn off all independent sources, leaving the dependent sources intact, as they are controlled by circuit variables. In this case we can proceed in two ways to find R_th:

        1. Apply a voltage source V₀ at terminals a and b and determine the resulting current i₀. Then R_th=V₀/i₀.

   2. Insert a current source i₀ at terminals a – b and find terminal voltage V₀. Again R_th= V₀/i₀.In either approach we may assume any value of V₀ and i₀.

        3. Considering the circuit mentioned above with independent sources; after finding R_th,  find the open circuit voltage V_oc which appears across the two terminals a – b. This is V_th.

        4. Replace the entire network by a single thevenin source, whose voltage is V_th or V_oc and internal resistance R_th or R.

        5. Connect R_L back to its terminals from where it was previously removed.

        6. Finally, calculate the current flowing through R_L, using the equation –

I = V_th/(R_th+R_L)   or

I = V_oc/(R_i+R_L)

To find V_th –

Using mesh analysis,

$-16+2{{i}_{1}}+12({{i}_{1}}-{{i}_{2}})=0$ And ${{i}_{2}}=-1A$

Solving for i₁, we get

$-16+2{{i}_{1}}+12{{i}_{1}}+12=0$ 

$14{{i}_{1}}=4$ $\Rightarrow {{i}_{1}}=\frac{4}{14}=\frac{2}{7}$ .

${{V}_{th}}=12({{i}_{1}}-{{i}_{2}})=12(\frac{2}{7}+1)=12\times \frac{9}{7}=15.42V$